WITHIN THIS FILE, YOU WILL SEE AN EARLY VERSION OF THE PUBLISHED ARTICLE: MARSON, S. HARRINGTON, C. & WALL, A. (2011). TEACHING STATISTICS TO BLIND STUDENTS. PRESENTED A THE 28TH ANNUAL BACCALAUREATE PROGRAM DIRECTORS CONFERENCE, APRIL 25 (CINCINNATI, OHIO). BECAUSE OF LIMITED SPACE FOR THE PUBLISHED VERSION, MUCH OF THE ORIGINAL MATERIAL WAS DELETED. THERE IS A GREAT DEAL OF USEFUL INFORMATION WITHIN THIS DOCUMENT THAT CAN ASSIST A STATISTICS PROFESSOR WHO HAS A STUDENT WITH A VISIONAL IMPAIRMENT.
Teaching Introduction to Statistics to Blind Students[1], [2]
Stephen M. Marson, Ph.D.
Department of Sociology and Criminal Justice
University of North Carolina at Pembroke
steve.marson@uncp.edu
Charles F. Harrington, PhD
Department of Management, Marketing, and International Business
The University of North Carolina at Pembroke
charles.harrington@uncp.edu
Adam C. Walls, MFA
Department of Art
University of North Carolina at Pembroke
adam.walls@uncp.edu
ABSTRACT
For social science majors who are not mathematically inclined, statistics is a challenging course. However once one can visualize a distribution, the course becomes more manageable. Consequently, blind students have a distinct disadvantage in learning basic statistical concepts. We address teaching strategies that create a more level playing field for blind students.
Key Terms: Statistics, Social Science Majors, Blind Students, Teaching, Visualization
Introduction
The statistics course is the most dreaded part of the curriculum for social science majors who have no visual impairment. Research supports this observation (Marson, 2007; Forte, 1995; Wilson & Rosenthal, 1992; Royse & Rompf, 1992). Teaching statistics to students with visional impairments or total blindness is exponentially more cumbersome than teaching statistics to sighted students (Gibson & Darron, 1999; Kapperman & Sticken, 2003; McCallister & Kennedy, 2001; Spindler, 2006). For a sighted person, understanding the fundamentals of statistics emerges with the basic appreciation of distributions. Therefore, an introduction to statistics courses begins with an initial objective of having students understand the normal distribution. Achieving this objective leads to the ability to understand inference. Gibson and Darron (1999) stress that in order to successfully understand basic statistical concepts; students must have “visual and spatial skills.” Later, they note, “Teaching statistics to students who are visually impaired is a unique challenge because instructors must find ways to convey the spatial and visual features of data sets and sampling distributions” (p. 130).
Statistics professors are beginning to admit that they have made the introduction to statistics courses more complicated than it needs to be (Yilmaz, 1996). For example, Stuart (1995) notes that introduction to statistics courses have been traditionally based on “mathematical requirements.” This is based on the premise that “the necessary mathematics must be in place before any real applications can be discussed.” He argues that, “not only is this unnecessary, it is counterproductive for most students” (p. 45). Later, he continues this line of thought (Stuart, 2003). In her research, Coulter (1995) notes that de-emphasizing mathematics provided a positive learning experience for students. The notion that we need to provide instruction in order to enable our students to be good consumers of statistical information lays the foundation for effective teaching to blind students.
The emphasis on teaching blind students (like sighted students) is an appreciation of statistics without stressing the “mathematical dimension.” The primary mission of this presentation is to demonstrate the use of high and low tech devices for the teaching of statistics to blind students. These include, but may not be limited to:
· Raised line drawing kits (commercially available) to sketch single system designs.
· Braille versions of distribution tables: z, t and F.
· 3-dimensional clay for a scatter plot for understanding a simple correlation.
· 3-dimensional z, chi and skewed distributions.
Blueprints and/or plans will be given to enable participants to replicate these teaching tools. The 3-dimensional z distribution will include pilings to represent the mean and 3 standard deviations on each side of the mean. The skewed distribution will include two pilings to represent the location of the mean and median. In addition to the technology aspects of the presentation, we will present specialized strategies for the oral presentation. A list of do’s and don’ts will be available.
Justification
If learning statistics includes such a high degree of frustration and nearly insurmountable obstacles for the blind student, is it worth the time and effort of the student and the professor? There is no doubt, that the struggle for the blind student is much greater than the sighted student. Anyone who has taught statistics has heard the question from sighted student: “Why do I have to take this course?” The answer is obvious to any statistics professor. One cannot make intelligent professional decisions without a fundamental knowledge of statistics. Fundamental knowledge of inference enables a professional (non-math person) to ask the right questions and see though fabrications that often emerge from our political structure. Greenfield (1993) offers the best-articulated justification for teaching statistics to an audience that requires a justification.
Review of Literature
The review of literature is divided into three parts. First, we offer a conceptual linkage between a student’s visual capacity and the severe limitations that vision places on learning math and statistical concepts. Second, efforts were made to seek out learning patterns for blind students. The identification of learning patterns will assist a professor in developing strategies for maximum learning with the least amount of stress for both the professor and student. Third, a discussion of countertransference is provided. Here we offer strategies for approaching a blind student.
The Visuality of Learning Statistics
One predominant and fundamental concept must be understood by every professor who is teaching statistics to blind students. The foundation for understanding math and statistical concepts is visual. This fact is undisputed (see Beck-Winchatz, 2008; Cahill & Linehan, 1996; Gibson & Darron 1999; Haas 1965; Landau, Russell, Gourgey, Erin, & Cowan, 2003; Liedtke, 1998; Meehan, Hoffert, & Hoffert, 1993; McCallister & Kennedy, 2001; Quek & McNeill 2006; Sahin, & Yorek, 2009; Spindler, 2006; Van Scoy, McLaughlin, Odom & Zuppuhaur, 2006). Two issues predominate. First, Liedtke and Werner (1998) provide clear evidence that fostering mathematics in children is a visual or concrete exercise. We learn basic arithmetic concepts by using our fingers. In the case of blind children, we feel our fingers to grasp arithmetic concepts. Thus, blind children are at a clear disadvantage – however, the disadvantage is not insurmountable. Second, even after one is able to comprehend basic arithmetic concepts, advanced learning in mathematics and statistics remains a visual enterprise. In addressing advanced learning, Penrose (1989, p. 424) writes, “...almost all my mathematical thinking is done visually.” Gestures made by professors while teaching advanced concepts can make the difference between understanding an abstraction or totally being lost. The clear conclusion is: Blind students with average to above average intelligence have a severe disadvantage.
Patterns in the Literature
A clear pattern emerges from the literature: A statistics professor has a blind student enrolled and does not know how to respond. The typicall one will review the literature to learn how to address the issues of teaching statistics to a blind student, but discover that very little has been published. As a result, the professor will conduct his/her own n=1 study and have the results published. All of these articles include a statement that notes, “there is very little literature on teaching statistics to blind students.” These articles (Andreou & Kotsis, 2005; Cahill & Linehan, 1996; Karshmer, Gupta, Geiger, & Weaver, 1999; McCallister & Kennedy, 2001; Meehan, Hoffert, & Hoffert 1993; Spindler, 2006) offer two dimensions of advancing our knowledge. First, the authors review the literature and typically list and/or describe tools that can be employed to advance statistical/mathematical knowledge of blind students. Second, they offer original findings that emerged from their work with an individual blind student or a small group of blind students. If a reader was attempting to find a pattern within this literature, one could easily conclude that the only common denominator among blind students is they cannot see. That is, the capacity to provide patterns of learning statistics among blind students is a Herculean task. However, following is a tentative list of issues that will assist statistics professors:
· It is rare to find a blind student who is not familiar with Nemeth Code. Nemeth Code, simply stated, is Braille for mathematics (for an introduction to Nemeth Code see: Kapperman & Sticken, 2003; Rosenblum & Amato, 2004). Over the past 15 years, efforts (Karshmer, Gupta, Geiger, & Weaver, 1999) have been made to develop software that can translate Nemeth Code (i.e. a blind student’s homework) into Latex Code; which is readable to the sighted professor). More recently, a professor, using Latex Code, can translate a homework assignment into Nemeth Code (Osterhaus, Weaver, Amerson, & Siller, 2001).
· There is no dispute: fundamental learning of math and statistics is visual. Based on this observation, blind students need to see these concepts with their hands. For example, how can one envision a normal distribution with standard deviations without being able to see a drawing of it? Three options for hand-seeing are available:
o One could construct a 3-dimensional normal distribution. This would give the student an opportunity to feel a curve and insert standard deviations in it. In this manner, the student could “see” the 68-95-99.7 rule.
o On a piece of wood, one could use a router and produce an indentation of a normal distribution. Indentations could be routed to represent standard deviations.
o One could use clay and form a normal distribution on a wood platform. Again, standard deviation could extend from the surface of the wood.
Although all three options afford the blind student an opportunity to “feel” a normal distribution, Landau, Russell, Gourgey, Erin, and Cowan (2003) suggest that the third option is best. Of the three options, the third is most like Braille. However, blind students (like sighted students), do not share a common learning strategy. For example, our student strongly preferred router style over the Braille style. Thus, it may not be a bad idea to include all three strategies within one’s teaching repertoire. “Which of the three strategies is best?” is an empirical question.
· Blindness severely limits a student’s ability to comprehend basic statistical concepts. Merely attending a statistics class and taking notes will not enable a blind student with above average intelligence to understand basic concepts. To create a level playing field, two options are available.
o Tutoring outside the classroom: Spindler (2006) demonstrates that tutoring is effective for a blind student enrolled in an advanced calculus class. The blind student must do work above and beyond those of sighted students. There are many problems associated with tutoring, but Spindler (2006) does an exceptionally good job of addressing them. The greatest concern for the tutor is the attitude of the professor toward the blind student.
o Independent Study Class: Although there is no literature that supports the use of independent study for teaching basic statistics to a blind student, administratively, the independent study is much less cumbersome and less expensive than tutoring. The amount of additional work required by the blind student is estimated to be between 1 and 2 credit hours. Ideally, a statistics lab credit would be the best option.
Both tutoring and the independent study would share the identical mission: to create a level playing field for learning statistical concepts.
· Two issues of test-taking for blind students is particularly noteworthy:
o Commonly, tests in introduction to statistics courses are open book and open notes. Initially, students are thrilled with open book/notes exam, until after they realize that the test answers can neither be found in the book or their notes![3] A wealth of research suggests that open book/notes for statistics tests offer great benefits to learning (Brightwell, Daniel, & Stewart, 2004; Kalish, 1958; Phillips, 2006; Williams & Wong, 2009). If sighted students are permitted to use notes and books, blind students must be afforded the same opportunity. The problem is the type of media use for notes and books. Without regard to the type of product used by the blind student, exam time must be extended and the student must be given the test alone.
o The conventional format for a statistics test is quasi essay. Typically, the professor will give a problem or a statement and the student will respond by illustrating his/her knowledge. The quasi essay format may be problematic for blind students. Quasi essay items have demonstrated to be overly fatiguing for a blind student (McCallister & Kennedy, 2001). Multiple choice items might be a better option for both the professor and student (Meehan, Hoffert, & Hoffert, 1993). Data indicate that multiple choice items produce less anxiety (Sommer & Sommer, 2009). Most importantly, multiple choice tests are not easier (Holtman, 2008) nor generate higher grades (Marson, 2007) than quasi essay.
· Textbooks are a serious problem for statistics courses – particularly when tests are open book/notes. Which kind of text is best: Braille or audio format? At first analysis, Braille seems like the best option. However, these Braille books are excessively expensive and too large to carry to class. In addition, Wessel, Smith and Rawles (1979) identified a trend in the decline of the use of Braille in the 1970s. Today, recent evidence suggests that less than 10% of blind people can read Braille (Boles, 2010) and that this trend is international (Papadopoulos & Koutsoklenis, 2009). What is the best format for a blind student to employ that will afford them the best avenue for understanding the various nuances associated with the introduction to inference? In conducting an intensive review of literature (with the assistance of four reference librarians), not a single article could be found. However, after several lengthy discussions with various professionals who specialize in educational services for blind students, one option appears to be best for the study of statistics: a statistics textbook that is offered in an audio format (formulas and graphics not included) in conjunction with formulas in Nemeth Code and graphics provided in tactile representation.
As statistics professors gain more experience in teaching blind students, more patterns are likely to emerge. It is hoped that the list of patterns outlined within this review of literature can be expanded.
Countertransference
Although much of Freud’s psychoanalytic theory has been discredited, he was able to produce many concepts that continue to have practical application today. The concept of countertransference can assist in understanding the development of teaching strategies for blind students. Within the context of this paper, countertransference constitutes a process by which a statistics professor engages in a role that violates the standard practice of interacting with a student. Traditionally, the concept of countertransference has been employed as a catalyst for articulating inappropriate sexual relationships. However, the concept includes any type of alternative role activity that a person of power accepts which misdirects a relationship from achieving a predetermined goal.
J. Saylor (Personal Communication, June 10, 2010) outlines three critical countertransference components that can prove harmful for the instruction of statistics to a blind student.
· Do not lie or exaggerate the blind student’s statistical competence.
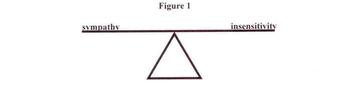
The statistics professor may feel sorry for the blind student. Feelings of sympathy lead to grade inflation. Grade inflation translates into students believing that they are more capable than their actually knowledge reflects. Some professors have interpreted the avoidance of sympathy as following a path of being impatient or intolerant of a student’s difficulty in understanding statistical concepts. As illustrated in Figure 1, a balance between sympathy and lacking the sensitivity of the difficulties of blindness is necessary.
Striking a balance between being overly sympathetic versus insensitivity is the key. Landau, Russell, Gourgey, Erin, and Cowan (2003) note that their focus group research clearly demonstrates that blind students profoundly resent faculty who give too much assistance. On the other hand, Spindler (2006) demonstrates that blind students can become hostile to professors who refuse to accommodate for blindness. In Spindler’s work, a professor teaching a fourth year calculus course continued to include statements like “as you see on the curve” even though he was told that such statements offered no assistance to blind students.
· Understand that the teaching of statistics must be conceptually linked to professional goals.
Statistics is a tool to achieve professional goals within a career track. Mills (2003) provides a conceptual framework based on empirical evidence that emphasizes the critical linkage of new knowledge to current ideas or new knowledge. More succinctly, the fertile soil for the acquisition of new statistical knowledge is the ability to link new ideas to practical problem-solving. Students (blind or sighted) have the greatest chance of understanding statistical concepts when such concepts are linked to a professional goal (Garfield, 1995; Garfield and Ben-Zvi, 2007; Cobb, 1992; Cobb and Moore, 1997). The notion that we need to provide instruction in order to enable our students to be good consumers of statistical information lays the foundation for effective teaching to blind students. During the beginning of the decade, numerous works focused on the listing of teaching priorities that should be established for comprehending statistics (these works include Moreno, 2002; Schield, 2002; Watson and Callingham, 2003). However, first and foremost, these priorities must be founded on the student’s major. Thus, as noted earlier, learning statistics for the blind student is a highly individualized and work-intensive enterprise for the professor.
· Know when to concede that the student will not be able to comprehend the material within a given timeframe.
Teaching introductory statistics is a highly visional enterprise. A blind college student with average intelligence will find learning to be cumbersome at best. When teaching blind students, it might be necessary to include lab time exclusively for the blind student. Here, the student can be given three-dimensional objects that represent normal distributions, standard deviations, skewed distributions, means and medians, etc. Although cumbersome to build, a Quincunx can be of great usefulness. Judgment of the professor is needed. Although college students must achieve course objectives within a defined time frame, the introductory statistics courses may need to be extended for the blind student. On the other hand, no student (sighted or blind) can be given an infinite amount of time to learn statistical concepts. A question must be asked which is based on the concepts introduced in the two bullets listed above: Is the statistical concept in which the blind student is unable to comprehend relevant to the student’s educational goals? If it isn’t, forcing the issue is counterproductive to the learning experience. If it is, the student must receive a failing grade.
Visual Tools for Teaching
The review of literature demonstrates that the fundamental understanding of statistical concepts emerge from visual illustrates. Without this visualization, the blind student will face a course that limits their ability to reach their full potential for understanding and applying statistical concepts. The most complex dimension of teaching statistics to a blind student is the reconstruction of images that professors employ for sighted students. Unfortunately, the typical statistics professor will not have the necessary skills to construct an accurate portrayal of statistical artifacts for the blind students.
Three particularly useful resources are available for professors. First, Inge Formenti from the American Printing House for the Blind lists a series of resources (see Appendix A). Any professor who is teaching statistics to a blind student should review this list.
Second, PIAF (Pictures In A Flash) is a machine that makes raised line drawings on special paper, called capsule or swell paper. Users can draw, print or photocopy pictures onto the swell paper and pass it through the Piaf. The heat of the Piaf causes the lines to swell and then the drawing can be read with the fingers. It is ideal for people who are blind and vision impaired. Piaf is being used in a variety of educational, employment and personal settings (Debbie Kay Lowery, Personal Communication, March 11, 2011). Features: • Production speed dependent upon complexity of graphic • Uses Single Sheet SwellTouch Paper, Maximum size up to A3 (11" x 7") • Audio Indicator lets you know when to insert the next sheet. • Special circuit to extend heater life • Energy efficient and quiet. • Strong, easy-grip handle and fold down paper trays. • Comprehensive safety features
Uses for PIAF: • Introduce simple shapes • Learn to draw and expressing oneself graphically • Learn about handwriting & signatures • Access graphical information for Math and Sciences • Create maps for orientation and mobility training • Make a chess board accessible • Explore world maps to identify countries & regions • Understand business charts and graphical data
Third, after a year of research, I discovered that the most efficient source for the construction of meaningful statistical artifacts is a campus’s Art Department. A professor who specializes in Sculpture has the greatest potential of creating the necessary artifacts for the blind student. Following are the directions for an Art professor to use for constructing the necessary artifact:
Producing a relief image of the bell curve
Using this method to transfer the image of a bell curve onto a wood board for rendering a relief image will require several supplies.
Before beginning this project you will need the following items
· Tracing paper or carbon paper
· Particle board or most any 8inch by 11 inch (or larger) wood board at a thickness of ½ inch or thicker
· Router
· Pencil
· Scanner/Printer
· Polyurethane
· Paint brush
· Sand paper
Steps
1. After finding the image that you want to transfer the image can be scanned and printed to a size of your choosing
2. You can either trace over this image with tracing paper, or simply place a sheet of carbon paper between the printed image and the board and use a pencil to rub the transfer ink onto the board
3. If you choose to use the tracing paper, you will have the additional step flipping the tracing paper back over after the first tracing to transfer the image accurately.
4. The board that you are transferring onto should be free of knots and have a smooth surface. Particle board or MDF (medium density fiberboard) may produce better results than a pine board since the particle board has no wood grain and will yield a smoother surface. In either case, you should avoid chip board and plywood due to their rough surfaces.
5. After the image is transferred, you will need to use a router with a ¼ inch diameter bit (or larger) set a depth of ¼ inch to cut away the image you have transferred onto the board. A little light sanding and a brushed on coat of polyurethane will help protect your fingers from the wood and the wood from your fingers.
Because of the controversy addressed in the review of literature, Mr. Frank Cummings, a visually impaired graduate of our university, assessed the quality of this tactile tool. Although counterintuitive, he preferred the “router option.” Because of his blindness, he was troubled by the concept of a 3-dimensional normal distribution and could not comment on the utility of it. A video is available of Mr. Cummings’ assessment by clicking on the picture within Video 1.
Video 1
Mr. Cummings confirmed the major theme stressed within the review of literature: The only thing that blind students have in common is their visual disability. Thus, a professor has a responsibility to construct teaching strategies based on the learning pattern inherit within the individual blind student.
Conclusions and Summery
Three major themes exist within this work. First, knowledge and application of statistical concepts are imperative for a literate citizenry (Moreno, 2002; Schield, 2002; Watson and Callingham, 2003). For example, knowledge of random sampling, confidence levels and confidence intervals are necessary for understanding news reports during election cycles. In addition, knowledge of statistics is a requirement within most professional and academic programs.
Second, comprehension of statistical knowledge is founded on a student’s ability to visualize statistical concepts (Beck-Winchatz, 2008; Cahill & Linehan, 1996; Gibson & Darron 1999; Haas 1965; Landau, Russell, Gourgey, Erin, & Cowan, 2003; Liedtke, 1998; Meehan, Hoffert, & Hoffert, 1993; McCallister & Kennedy, 2001; Quek& McNeill 2006; Penrose, 1989; Sahin, & Yorek, 2009; Spindler, 2006; Van Scoy, McLaughlin, Odom & Zuppuhaur, 2006). Thus, blind students with average to above average intelligence are severely limited in their ability to compete with sighted students in acquiring a statistical education.
Third, professors who are involved in teaching statistics have an ethical responsibility to create a level playing field for visually impaired students. The statistics professor must consider two major responsibilities. First, a professor needs to offer a balance between sympathy and insensitivity. Professors may not be overly sympathetic nor may they be insensitive to the special needs of a student. The literature demonstrates that serious problems emerge in an unbalanced teaching environment (Landau, Russell, Gourgey, Erin, and Cowan, 2003; Spindler, 2006). Second, constructing a series of statistical artifacts is the best avenue to instill basic statistical concepts for the visually impaired student.
References
Andreou, Y., & Kotsis, K. (2005). Mathematical concept development in blind and sighted children. International Journal of Learning, 12(7), 255-260.
Beck-Winchatz, B. (2008). Advancing participation of blind students in science, technology, engineering and math. Advances in Space Research, 42(11), 1855-1858.
Boles, D. W. (2010). The McSweeney’s Small Chair Review. Retrieved July 29, 2010, from Memeingful, Wordpress.com. Web site: http://memeingful.com/2010/02/16/as-braille-dots-fade-blind-illiteracy-builds/.
Brightwell, R., Daniel, J. H., & Stewart, A. (2004). Evaluation: Is an open book examination easier? Bioscience Education E-Journal, 3, Retrieved from http://bio.ltsn.ac.uk/journal/voln/beej-3.3.htm.
Cahill, H. & Linehan, C. (1996). Blind and partially sighted students' access to mathematics and computer technology in Ireland and Belgium. Journal of Visual Impairment and Blindness, 90(2), 105-112.
Cobb, G. W. (1992). Teaching statistics in L.A. Steen (ed) Heeding the Call for Change: Suggestions for Curricular Actions, MAA Notes 22, Washington, DC: Mathematical Association of America
Cobb, G. W., & Moore, D. S. (1997). Mathematics, statistics, and teaching. American Mathematical Monthly, 104(9), 801-823.
Coulter, X. (1995). A new way of teaching statistics. Journal of Educational Technology Systems, 24 (2): 181-187
Forte, J. (1995). Teaching statistics without sadistics. Journal of Social Work Education, 31 (2): 204-218.
Garfield, J. (1995). How students learn statistics. International Statistical Review, 63(1), 25-34.
Garfield, J., & Ben-Zvi, D. (2007). How students learn statistics revisited: A current review of research on teaching and learning statistics. International Statistical Review, 75(3), 372-392.
Gibson, W., & Darron, C. (1999). Teaching statistics to a student who is blind. Teaching of Psychology, 26(2), 130-131.
Greenfield, T. (1993). Communicating statistics. Journal of the Royal Statistical Society, 156(2), 287-297.
Haas, V. (1965). The use of number rods in mathematics. Exceptional Children, 31(6), 297-300.
Heijne-Penninga, M., Kuks, J. B., Schonrock-Adema, J., Snijdersm, T. A. & Cohen-Schotanus, J. (2006). Open-book tests to complement assessment-programmes: Analysis of open and closed-book tests. Advances in Health Sciences Education, 13, 263-273.
Holtzman, M. (2008). Demystifying application-based multiple-choice questions. College Teaching, 56(2), 114-120.
Kalish, R. (1958). A experimental evaluation of the open book examination. Journal of Educational Psychology, 49(4), 200-204.
Kapperman, G., & Sticken, J. (2003). A case for increased training in the Nemeth Code of braille mathematics for teachers of students who are visually impaired. Journal of Visual Impairment & Blindness, 97(2), 110-112.
Karshmer, A. I., Gupta, G., Geiger, S., & Weaver, C. (1999). Reading and writing mathematics: The MAVIS Project. Behaviour & Information Technology, 18(1), 2-10.
Landau, S., Russell, M., Gourgey, K., Erin, J., & Cowan, J. (2003). Use of the talking tactile tablet in mathematics testing. Journal of Visual Impairment & Blindness, 97(2), 85-96.
Liedtke, W. & Werner (1998). Fostering the development of number sense in young children who are blind. Journal of Visual Impairment and Blindness, 92(3), 346-349.
Marriott, J., Davies, N., & Gibson, L. (2003). Teaching, Learning and Assessing Statistical Problem Solving. Journal of Statistics Education, 17(1). Retrieved from ERIC (EJ841530).
Teaching, learning and assessing statistical problem solving. Journal of Statistics Education, 17(1). Retrieved from ERIC (EJ841530).
Marson, S. (2007). Three empirical strategies for teaching statistics. The Journal of Teaching Social Work, 27, (3-4) 199-213.
Meehan, A. M., Hoffert, D., & Hoffert, L. L. (1993). Strategies and resources for teaching statistics to visually impaired students. Teaching of Psychology, 20(4), 242-244.
McCallister, C. J. & Kennedy, R. L. (2001). Teaching statistics to a student who is blind. Presented at the Annual Meeting of the Mid-south Educational Research Association, November 16 (Little Rock, AR).
Mills, J. D. (2003). A theoretical framework for teaching statistics. Teaching Statistics, 25(2), 56-58.
Moreno, J. E. (2002). Toward a statistically literate citizenry: What statistics everyone should know. Presented at the 6th annual International Conference on Teaching Statistics, July 7-12 (Cape Town, South Africa).
Quek, F., & McNeill, D. (2006). embodiment awareness, mathematics discourse, and the blind. Annals of the New York Academy of Sciences, 1093(1), 266-279.
Osterhaus, S.A., Weaver, C., Amerson, M., & Siller, M.A. (2001). More accessibility for math students: AFB Solutions Forum stakeholders and their pursuit of braille conversion software. Journal of Visual Impairment & Blindness, 95(3), 184-188.
Penrose, R. (1989). Emperor's New Mind. London: Random House.
Phillips, G. (2006). Using open-book tests for strengthening the study skills of community-college biology students. Journal of Adolescent and Adult Literacy, 49(7), 574-582.
Phiri, P. A. (1993). A comparison of assessment by closed book and open book tests. International Journal of Education Science Technology, 24(1), 23-26.
Papadopoulos, K. & Koutsoklenis, A. (2009). Reading media used by higher-education students and graduates with visual impairments in Greece. Journal of Visual Impairment and Blindness, 103(11), 772-777.
Rosenblum, L., & Amato, S. (2004). Preparation in and use of the Nemeth Braille Code for mathematics by teachers of students with visual impairments. Journal of Visual Impairment & Blindness, 98(8), 484-495.
Royce, D. & Rompt, E.L. (1992). Math anxiety: A comparison of social work and non-social work students. Journal of Social Work Education, 28 (3), 270-277.
Sahin, M., & Yorek, N. (2009). Teaching science to visually impaired students: A small-scale qualitative study. US-China Education Review, 6(4), 19-26.
Schield, M. (2002). Three kinds of statistical literacy: What should we teach? Presented at the 6th annual International Conference on Teaching Statistics, July 7-12 (Cape Town, South Africa).
Smith, D. (2006). Developing mathematical concepts through orientation and mobility. Re:View, 37(4), 161-165.
Sommer, R. & Sommer, B. (2009). The dreaded essay exam. Teaching of Psychology, 36, 197-199.
Spindler, R. (2006). Teaching mathematics to a student who is blind. Teaching Mathematics & its Applications, 25(3), 120-126.
Stuart, M. (1995). Changing the teaching of statistics. The Statistician, 44 (1): 45-54.
Stuart, M. (2003). An Introduction to Statistical Analysis for Business and Industry – a Problem Solving Approach. London, AL: Hodder Arnold.
Van Scoy, A. F., McLaughlin, D., Odom, J. V., & Zuppuhaur, M. E. (2006). Touch mathematics: A prototype tool for teaching pre-calculus to visually impaired students. Journal of Modern Optic, 53(9), 1287-1294.
Vyas, G. & Vyas, J. G. (2009). A comparative study of open book exam and closed book exams. International Research Journal, 2(7), 164-165.
Watson , J. & Callingham, R. (2003). Statistical literacy: A complex hierarchical construct. Statistics Education Research Journal, 2(2), 3-37.
Weber, L. J., McBee,, J. K., & Krebs, J. E. (1983). Take home tests: An experimental study. Research in Higher Education, 18(2), 473-483.
Wessells, M. B., Smith, A. E., & Rawles, B. A. (1979). A Study of Braille and talking book program in Ohio. Columbus, Ohio: Battelle Memorial Institute.
Williams, J. B. & Wong, A. (2009). The efficacy of final examinations: A comparative study of closed-book, invigilated exams and open-book, open web exams. British Journal of Educational Technology, 40(2), 227-236.
Wilson, W. C. & Rosenthal, B. S. (1992). Anxiety and performance in an MSW research and statistics course. Journal of Teaching in Social Work, 6 (2): 75-85.
Yilmaz, M. R. (1996). The challenge of teaching statistics to non-specialists. Journal of Statistics Education, 4 (1). http://www.amstat.org/publications/jse/v4n1/yilmaz.html
Appendix
Prepared by Inge Formenti, MLS
American Printing House for the Blind
Math Teaching/Learning Products
· http://www.aph.org/tgil/ database of images for tactile graphics
Textbook sources
· www.accesstext.org textbook file source for text to speech, braille production, etc.
Miscellaneous Links
· http://s22318.tsbvi.edu/mathproject/ch3.asp#main nice insights
· http://www.blindscience.org/ncbys/About_the_NCBYS.asp an organization directly concerned with teaching math to blind students
· http://ncam.wgbh.org/experience_learn/educational_media/stemdxaudio description
· http://ncam.wgbh.org/experience_learn/educational_media/stemdx/math_equations
· www.accesstext.org textbook file source for text to speech, braille production, etc.
· http://www.purdue.edu/odos/drc/Tactile_Diagram_Manual_PRINT.pdfPurdue’s take on tactile graphics—they used to sell a CD of tactile graphics, images, although I am not sure if that is still the case
[1] This work was originally presented at the 28th Annual Baccalaureate Program Directors Conference, April 25, 2011 (Cincinnati, Ohio).
[2] By the term “blind,” we are not referring to “legal blindness” or “vision impairment.” We operationally define “blind” as “no functional vision.” That is unable to read print.
[3] On tests, I have included the page number for the formula needed to solve the problem. Even armed with this information, it is common for students to get the test item wrong.